Extended Analysis of Functions 2

Summer/Fall 2006

Schedule

Assignments and classes will run according to this schedule. Please let me know if any of these dates conflict with a university-recognized religious holiday that you observe as a matter of faith.

All assignments must be submitted in the form of typed, word processor documents (MS Word) unless otherwise noted in the assignment schedule or the assignment itself. Upload elecronically-submitted assignments to your private page on the EAF 2 Course Forum.

You might also wish to consult lesson logics, discussions, and assignments listed in the Exended Analysis of Functions 1 website.

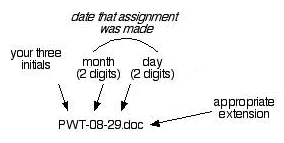
Please name your assignment files by the convention given below (files not following this convention will be returned unread). Name the file with:

• Your three initials
• The month (two digits) and day (two digits) that the assignment appears in the schedule (even if it differs from the date it actually is made)
• "-1", "-2", etc. if your submission is in two or more parts (which might happen when you want to send graphs or GSP sketches).
• an appropriate extension (this typically is attached automatically by your operating system. I'll tell you whether you need to include it manually after receiving your first submission ).

Date	In Class	Assignment
Jun 5	Introductions Questionnaire Overview Covariation as a foundational mathematical idea What is speed? What is speed at an instant? How would you measure it? Problem in understanding "instantaneous speed" Constant speed Average speed	Course Forum: Discuss why the idea of "instantaneous speed" should be problematic for students. Course Forum: Discuss the definition of average rate of change that was given today.
Jun 6	Graphing points and vectors in GC. Interpreting constant rate of change Linear functions and proportionality (mowing lawns, etc) Average speed as a function Graphing average speed functions	Average rate of change assignment (submit to your personal page at Course Forum) See calculus students comments Suppose g(x) is a function defined for all real numbers. What about g(x) is defined by the function f(x) = g(x)/x? Play with graphs of f for various definitions of g. Answer these questions.
Jun 7	Average rate of change of a function Average rate of change as a function Defining tangents to curves using rates of change Uses of parameters in GC to show relationships within a graph Introduce parametric functions Introduce parametric functions in 3-d Curves on a surface	Parametric functions in 2-d Paramteric functions in 3-d
Jun 8	Parameters vs parameters Parametric functions (again) Designing aids to help students "see" functions in 3-dimensions Test 1 (In Class; Take Home)	Do Take Home exam.
Jun 12	Discuss high school students working with curves in 3d--what is possible? Definition of a plane in 3-space What functions have planes as their graphs? Rate of change on a surface ... What does this mean? Simple cases	Course Forum: Continue discussion of under what conditions and to what benefit might we include ideas of graphs and surfaces in 3d in the high school math. curriculum Course Forum: Give your thoughts on the aims and purposes of the 6/12 lesson on functions that have planes as graphs.
Jun 13	Tangent lines to a surface Tangent planes to a surface Create a GC document that, given any function f(x,y) and a point on f's graph, produces tangent lines to the graph of f in the x- and y- directions and produces the plane containing those tangent lines.	Explain the development of your formulas. Assume you are explaining them to a student who knows how to use GC, understands that any function that has a plane as its graph is of the form f(x,y) = nx + my + K (and understands why this is so), and understands that a function g having a rate of change of m with respect to its argument implies that however much the function's argument changes, the functions value changes m times as much. (If I've omited anything that is important to your explanation, then include it and say what it is they understand about it.) Post your explanation to your personal page at Course Forum
Jun 14	More on averate rate of change Lines and curves -- linear approximations "Smoothness" of a function Issues of smoothness Accumulations and Riemann Sums Focus on why some Riemann sums are step functions and why some are not More on Riemann Sums	Modeling accumulative quantities as mathematical functions
Jun 15	Accumulations and rates of change--how fast does an accumulating quantity accumulate? Fundamental Theorem of Calculus Test 2	Prepare for Fall 06
Aug 21	Discuss the nature of explanations (examples from analysis and from geometry) Geometric constructions (compass and straightedge) What makes a figure a geometric figure? Activity Discussion of constraints and dependencies Introduction to Sketchpad 4.0 Points Straight things Circles More What does a proof prove? Whence "the thing to be proved"? Fundamental constructions	Constructions 1 (See discussion of difference between making a duplicate segment and making a duplicate segment given a segment, a line, a point on the line, and having that point as an endpoint) Saturday Math Club
Sep 11	Dependencies and assumptions Assumptions, conjecures, and arguments	Constructions 2 (with hints) See sample work with comments
Sep 25	Geomery and algebra Using Sketchpad as a teaching aid in algebra and calculus	Examine problems from Functions 1, Modeling. Pick two problems (other than #1); create a dynamic illustration that you could use with an overhead projector to help students understand the problem. Pick 3 problems from additional constructions. For construction problems, develop a goal structure for the construction and create the construction.
Oct 9
Oct 23
Nov 13
Nov 27
Dec 11
	Present projects Party at Dr. T's home, 6:00p